Mathematics 1

Topic outline

• Select topic General

  General

  Collapse all Expand all
  • Select activity Announcements

    

    Announcements Forum
• Select topic Definition of scale

  Definition of scale

  

  1- Information about the scale
  univ - Djilali bou naama			university
  Faculty of Economic, Commercial and Management Sciences.			college
  Common torso			section
  first year.			level
  Mathematics 1			The scheduled scale
  methodology.			Education Unit
  Lecture + oriented works			Lesson type
  first			hexagon
  02.			coefficient
  04			credit
  3 hours	Courier size per week	14 weeks in the first hexagon about 42 hours	Courier Size
  an hour and a half	Lecture
  an hour and a half	oriented works
  All your concerns about the lecture or business addressed via professional email will be answered within 48 hours.	nadjet.benfreiha@univ-dbkm.dz	e-mail	Communication Window

  The math scale is one of the methodological teaching modules of the composition presentations in the LMD system Study in the first six years of the first year Bachelor of Common Trunk, Mathematics is a set of abstract knowledge resulting from logical conclusions applied to groups. quantitative topics, helping us to understand, compare and understand the world around us and develop the ability to think properly and reasonably, Mathematics is involved in many other fields such as technology and science, helping researchers and scholars find solutions to problems and make the right decisions.

  Mathematics helps provide logical solutions and address everyday issues such as project planning, project management, budgets and even discussion management.
• Select topic Evaluation method

  Evaluation method

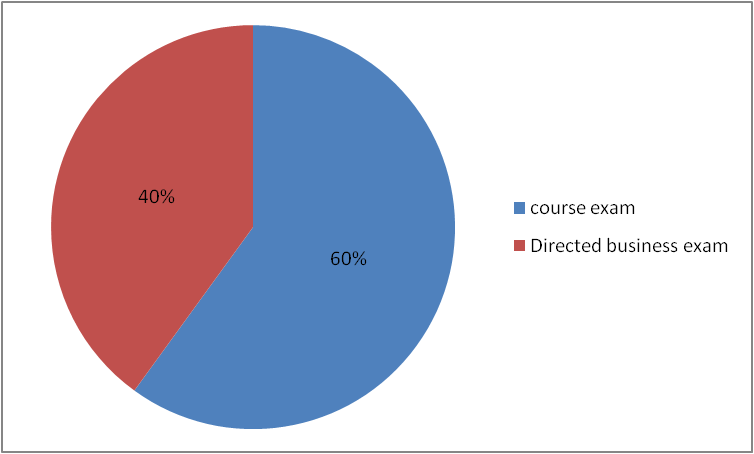
  To pass this subject, you must obtain a grade greater than or equal to 10 out of 20. If this is not possible, you can take the remedial course exam, where the course grade is calculated through the regular course exam, which represents 60%, and the directed work point, which represents 40%.

  
• Select topic contact info

  contact info

  

  Professor Benfreiha Najat

  Department of Commercial Sciences, Faculty of Economic and Commercial Sciences and Management Sciences, Djilali bou naama University, Khamis Milaina

  Email:

  All your concerns about the lecture or business addressed via professional email are answered within 48 hours.
• Select topic Communication Space

  Communication Space
  • Select activity Student Forum

    

    Student Forum

    Completion

    Students must

    

    Mark as done

    

  • Select activity Chat and Conversation

    

    Chat and Conversation

    Completion

    Students must

    

    Mark as done

    

  • Select activity wiki

    

    wiki

    Completion

    Students must

    

    Mark as done

    

• Select topic Scale Objectives:

  Scale Objectives:

  

  At the end of this measure, the student must be able to identify, interpret, discuss and conclude. The objectives of this measure are:

  •   Get to know what math is.

  •   Enabling students to be empowered in the fields of research, interpretation and ability to make sound decisions.

  •   Identify quantitative methods used in data processing and anticipate possibilities.

  •   The student has a clear vision of the most important aspects of mathematics that enable him to work in different fields in life such as economy and trade.

  •   Enabling students to deal with methods of thinking and forming and developing sound mathematical trends.

  •   Accurate and correct understanding of the most important axes of mathematics.

  •   To provide students with a range of experiences in mathematics to help them present the results of qualitative economic research in a specific quantitative, clear, concise and accurate manner.

  Through this class we will try to present math 1, in a simplified and clear manner, where: upon completion of this axis the student will be familiar with the objectives of the axis based on Bloom's cognitive levels:

  1. Level of knowledge and memory: Students at this level recover information from memory (Tribal gains), where students save definitions associated with math theme 1, acquire the necessary skills that enable them to use optimal methods of counting and analysis that help them to view data. Students are given multiple choice questions, asked to answer them and can be given empty filling questions, with the aim of conjuring their tribal gains related to mathematics.

  2. Level of assimilation and understanding: The student clarifies the basic characteristics that allow him to review the various variables and concepts in the axis. Here, the student gives some varied questions based on what has been used and understood for the study.

  3. Application Level: Students learn about various concepts related to Mathematics 1, use this on quantitative measurements of different economic phenomena and interpret them, and ask students to clarify the concepts by providing relevant examples of reality and working life.

  4. Level of analysis: Students distinguish between statistical methods of work in field studies, having the student study and analyze the most important possible theories.

  5. Level of Installation and Creation: Students search for the role of mathematics in various economic activities through careful examination, and here students brainstorm to find the causes of the problem and how to solve it based on the benefits of the lectures provided.

  6. Calendar level: The student studies compromise analysis and numerical and geometric sequences as well as logarithmic and core functions based on the sources and tools available to him and measuring them. We develop a final exercise in which the various processes are determined about what has been addressed in the scale.
• Select topic Tribal gains:

  Tribal gains:

  

  This article requires prior knowledge from the student about the concept of probability theory and the basic methods of the number in addition to his good knowledge of the mathematical aspect. A good understanding of the quantitative, computational and mathematical methods preceding this article, in order for the student to absorb this measure, must be aware of: Quantitative theories, probabilities, dals and derivatives, this will make it easier for the student to conclude or appreciate to solve problems and help make decisions. The student may also have to expand his cognitive interests to reveal the added value of what he learns about the mathematics scale.

  All who study mathematics should acquire and possess many experiences, skills and abilities, which allow you to have good control over the scale, among which are:

  - Desire for self-development and diligence in specialization.

  - Mathematical and computational skills.

  - Acquaintance with various mathematical concepts and terminology related to mathematics.

  • Select activity Test tribal gains:

    

    Test tribal gains: Choice

    Completion

    Students must

    

    Mark as done
• Select topic lesson plan

  lesson plan

  

  Your dear students can view the lesson plan through the following file:
• Select topic Introduction:

  Introduction:

  

  Mathematics is a study primarily related to representation and inference about abstract objects such as enemies and spaces, mainly used in many fields such as nature science, engineering, medicine and several others, as well as developing new disciplines in mathematics such as statistics and therefore there is no line between theoretical and applied mathematics.

  The mathematics scale is for first-year Bachelor's students, and has been divided into five themes, including 20 heartbreaking classes over 14 weeks. The first focus was on giving the student the most basic principles that help the student to enter the depth of this scale. It included concepts about compromise analysis by introducing the factor and addressing the most important methods of counting, and then identifying the arrangements, conciliations and characteristics of the student.

  Umm Al-Haq II included generalizations about numerical sequences by introducing general concepts and then addressing the computational sequences and their laws, engineering sequences and laws while applying the state of savings with composite interest.

  For the third axis, it included logartmatic and exponential functions, where we will provide a definition of the function and what the definition set is with applications of certain functions. We will also address the definition of both the logartmatic and essential function and its computational and analytical properties.

  In Axis IV, we will address derivatives through the corporal of derivatives and derivative laws, and its application to the concept of the great and small threshold value.

  Through the fifth axis we will learn about the original functions and calculation of integration by introducing basic concepts about the original functions, limited and unlimited integration and its characteristics, as well as applications of integration in the economic sciences.
• Select topic mind map

  mind map

  The following map is a detailed explanation of the mathematics scale for first-year students.

  

   
• Select topic lesson plan

  lesson plan

  

  Dear student you can view the lesson chart from the attached file.

  • Select activity lesson plan

    

    lesson plan File

    Completion

    Students must

    

    Mark as done
• Select topic Introduction to Axis I: Compromise Analysis

  Introduction to Axis I: Compromise Analysis

  

  The analysis is interested in giving the number of possible ways for groups under certain conditions through some sports rules that facilitate this composition on the one hand and enable the study of the completed groups by simplifying their counting and devising more effective methods more effective the number of appropriate cases and the number of possible cases associated with that incident on the other and thus the calculation of probabilities becomes one of the most important practical applications of compromise analysis, It is therefore obvious that these mathematical rules should be presented for use when addressing the topic of probability and its laws.

  In this chapter we will try to address the following topics:

  ·         Exchange

  ·         Arrangement

  ·         Harmony

   

  

  Through this chapter we will try to provide compromise analysis, in a simplified and clear manner, where: upon completion of this axis the student will be familiar with the objectives of the axis based on Bloom's knowledge levels:

   

  1.         Level of knowledge and remembrance: Students at this level recover information from memory (tribal gains), where students save definitions associated with the subject of mathematics, acquire the necessary skills that enable them to use optimal methods of counting and the student is given multiple choice questions, and is asked to answer them.

  2.         Level of assimilation and understanding: The student clarifies the basic characteristics that allow him to review the various theories and concepts in the axis. Here, the student gives some varied questions based on what has been used and understood for the study.

  3.         Level of application: Students learn about different concepts of compromise analysis, and use this to quantify and interpret different economic phenomena, and ask students to clarify the concepts by providing relevant examples of reality and working life.

  4.         Level of Analysis: Students distinguish between field working methods, having the student study the most important methods of dissemination.

  5.         Level of installation and construction: Students look for the role of compromise analysis in various economic activities through careful examination, and here students brainstorm to find the causes of the problem and how to solve it from the benefits of the lectures provided.

  6.         Calendar level: Students study the concepts of arrangements and conciliations, based on the resources and tools available to them and measure them. We develop a final exercise in which the various processes around the axis are determined.
• Select topic First axis: entrance to preview distribution

  First axis: entrance to preview distribution

  First axis: entrance to preview distribution
  Before addressing these topics, reference must be made to a fundamental point that constitutes the true basis of compromise analysis, namely the rule of multiplication and the rule of plural or the so-called basic principle of counting.
  First: Basic rules for methods of counting:
  There are two basic rules for the counting methods: the beating base and the collection base, which we summarize as follows:
  1.    Rule of beating:
  If there is a process or experience consisting of a number of K stages so that:
  -    The first phase takes place in a number of n1 different ways
  -    The second stage takes place in a number of n2 different ways.
  -    .... And so
  -    The last stage K takes place in a number of nk different ways.
  The process as a whole can be carried out in a number of possible ways:

  n = n ´ n ´.......´ nk

  • Select activity Chat Room

    

    Chat Room

    Completion

    Students must

    

    Mark as done

    

  • Select activity forum

    

    forum

    Completion

    Students must

    

    Mark as done

    

  • Select activity Content of the first axis

    

    Content of the first axis File

    Completion

    Students must

    

    Mark as done

    

    Dear student  Upload the file and read it well

  • Select activity Oriented Business Series

    

    Oriented Business Series File

    Completion

    Students must

    

    Mark as done
  • Select activity First Axis Questions Test

    

    First Axis Questions Test Quiz

    Completion

    Students must

    

    Mark as done

    

    In case you are unable to solve exercises, you should review the lesson and reapply examples.

     

  • Select activity work shop

    

    work shop Workshop

    Completion

    Students must

    

    Mark as done

    

    Dear Student Choose One Exercise from the Series of Directed Works 1 and Solve It

• Select topic Introduction to the second axis: Generalities of numerical sequences

  Introduction to the second axis: Generalities of numerical sequences

  n the previous chapter, we examined the conciliation analysis, the definition of factors and the treatment of arrangements and conciliations. This chapter aims at the generalities of numerical successions.

  

  Once completed, the student will be familiar with the objectives of the hub based on Bloom's knowledge levels:
  1.    Level of knowledge and memory: Students at this level recover and organize information from memory (Tribal gains), where students learn about the concept of cascades, acquire the necessary skills that enable them to use optimal methods to calculate arithmetic and engineering sequences. Students are given multiple choice questions, asked to answer them and can be given questions to fill in empties, with the aim of restoring their tribal gains related to the axis.

  2.    Level of assimilation and understanding: The student identifies and identifies various variables and concepts related to the second axis of numerical sequences. Here, we give the student some varied questions based on what has been used and evaluated for the study.
  3.    Application level: The student can perform processes to identify the laws of the successive with the application of the savings status with a composite interest.
  4.    Level of analysis: The student distinguishes various types of computational and engineering sequences.
  5.    Composition level: Students organize information in different ways through brainstorming.
  6.    Calendar Level: The student evaluates and evaluates all information gained to make decisions based on the established criteria. We develop a final exercise in which the various processes of numerical successions are determined.
• Select topic Theme II: General numerical sequences

  Theme II: General numerical sequences

  Sequences:
  Definition: We call successive numerical each un application from N (or part of N) towards R, we usually symbolize the succession by Un or abbreviated (Un) the Un limit is called the general limit of the succession (Un)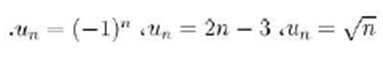

  

  • Select activity chat

    

    chat

    Completion

    Students must

    

    Mark as done

    

  • Select activity chat room

    

    chat room Forum

    Completion

    Students must

    

    Mark as done

    

  • Select activity numerical sequences

    

    numerical sequences File

    Completion

    Students must

    

    Mark as done

    

    Dear student upload the above file and read it well.

  • Select activity Second Axis Questions Test

    

    Second Axis Questions Test Quiz

    Completion

    Students must

    

    Mark as done

    

    Dear student if you cannot solve exercises you have to re-read the lesson and apply examples.

  • Select activity serie N2

    

    serie N2 File

    Completion

    Students must

    

    Mark as done

    

  • Select activity Workshop 2

    

    Workshop 2

    Completion

    Students must

    

    Mark as done

    

    Dear student choose one exercise from the second series and solve it.

• Select topic Axis III: Logarithmic and Asian functions

  Axis III: Logarithmic and Asian functions

  
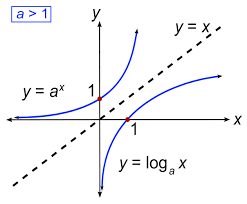
  Both exponential and logarithmic functions are used to describe many applications in our lives, such as their use in the sphere of population growth and changes in investments and their value. The mathematical relationship between exponential and logarithmic functions is reverse.
  As we have studied in the second axis numerical successions (arithmetic and engineering) and the definition of the generalities and laws of the numerical succession with the application of the savings situation, the objective of this axis is the generalities of logartamatic and exponential functions.

  

  
  Once completed, the student will be familiar with the objectives of the hub based on Bloom's knowledge levels:
  1.    Level of Knowledge and Remembrance: Students at this level recover and organize information from memory (tribal gains), where students learn about the concept of logartic and exponential functions, and acquire the necessary skills that enable them to use optimal functions. Students are given multiple choice questions, asked to answer them and can be given questions to fill in empties, with the aim of restoring their tribal gains related to the axis.
  2.    Level of assimilation and understanding: The student identifies and assigns various variables and concepts related to the third axis of Logartamian and Exponential departments. Here, we give the student some varied questions based on what has been used and evaluated for the study.
  3.    Application Level: The student can perform operations to identify the functions applications and their computational characteristics.
  4.    Level of Analysis: The student distinguishes different types of logartmatic and exponential functions and resolves equations and swings.
  5.    Composition level: Students organize information in different ways through brainstorming.
  6.    Calendar level: The student evaluates all the information gained and evaluates it to make decisions based on the specific criteria. We develop a final exercise in which the various functions are determined
• Select topic Generalizations about functions:

  Generalizations about functions:

  
  Definition: The function with a real variable is an application from the field of I towards R, we usually symbolize the function with f , and for the group of functions with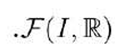
  The group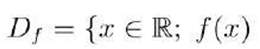 is the definition group of the function f .
  The group is the statement of function f.

  • Select activity chat room

    

    chat room Forum

    Completion

    Students must

    

    Mark as done

    

  • Select activity chat

    

    chat

    Completion

    Students must

    

    Mark as done

    

  • Select activity Third axis content

    

    Third axis content File

    Completion

    Students must

    

    Mark as done

    

    Dear student upload the above file and read it well.

  • Select activity Third Axis Questions Test

    

    Third Axis Questions Test Quiz

    Completion

    Students must

    

    Mark as done

    

    Dear student if you cannot solve exercises you have to re-read the lesson and apply examples.

  • Select activity Oriented Business Series 3

    

    Oriented Business Series 3 File

    Completion

    Students must

    

    Mark as done

    

• Select topic Axis IV: Derivatives

  Axis IV: Derivatives

  

  
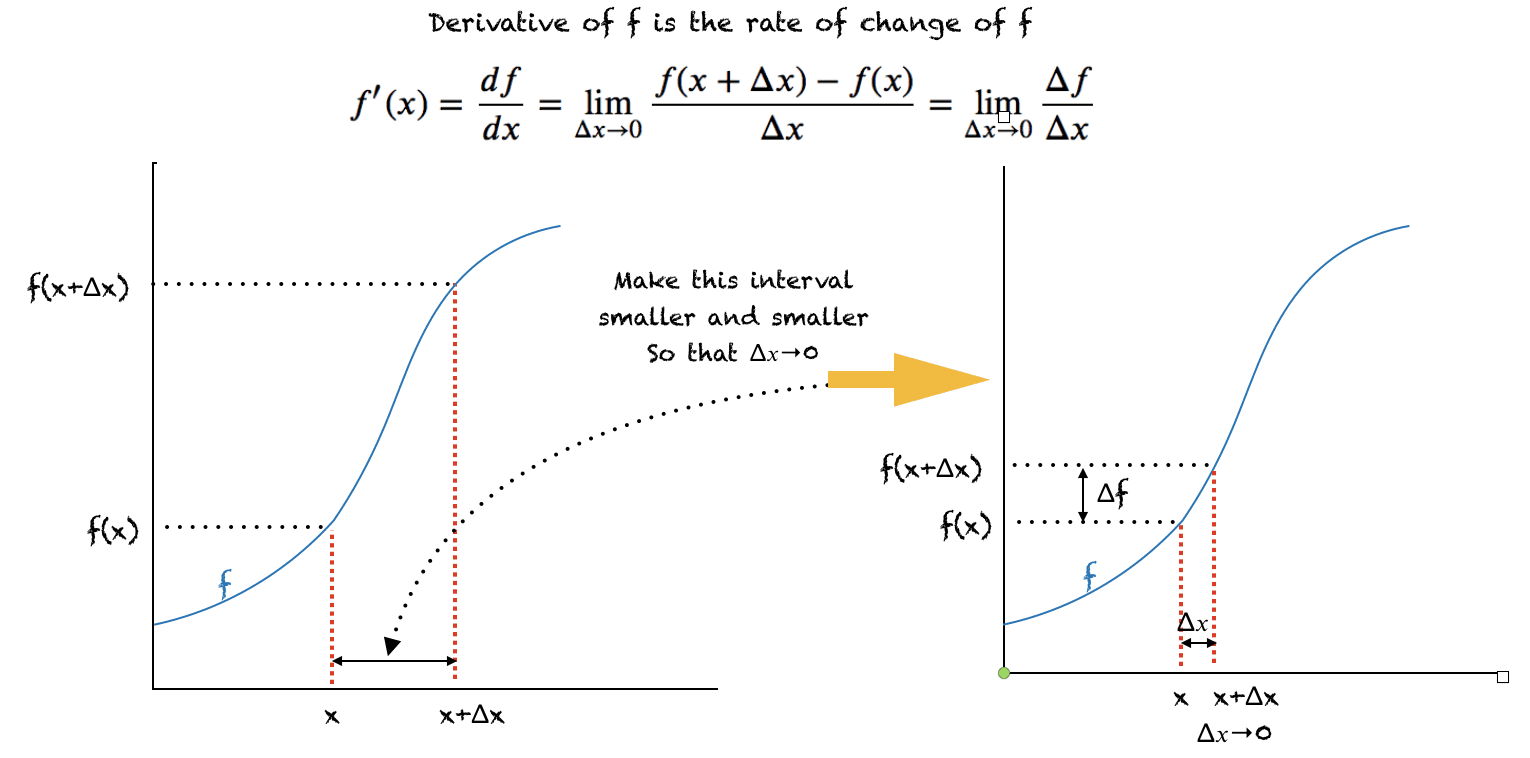
  Derivatives in mathematics are defined as the rate of momentary change in the function relative to a variable of its variables, and the process of finding the derivative is called differentiation, and the derivative is the inclination of the function's graph or the inclination of the tangent line at a certain point on it.

  

  Once completed, the student will be familiar with the objectives of the hub based on Bloom's knowledge levels:
  1.    Level of knowledge and memory: Students at this level recover and organize information from memory (Tribal gains), where students learn about the concept of derivatives and their laws, acquire the necessary skills to enable them to apply derivatives to the concept of great and small threshold value. Students are given multiple choice questions, asked to answer them and can be given empty filling questions, aimed at restoring their tribal gains related to the axis.
  2.    Level of assimilation and understanding: The student identifies and identifies various variables and concepts related to the fourth axis of derivatives. Here, we give the student some varied questions based on what has been used and evaluated for the study.
  3.    Application level: The student can perform derivation processes and learn about his laws. Level of analysis: The student distinguishes various processes on derivatives.
  4.    Composition level: Students organize information in different ways through brainstorming.
  5.    Calendar level: The student evaluates all the information gained and evaluates it to make decisions based on the specific criteria. We develop a final exercise in which the various derivatives processes are determined

  • Select activity chat

    

    chat Forum

    Completion

    Students must

    

    Mark as done

    

  • Select activity chatroom

    

    chatroom

    Completion

    Students must

    

    Mark as done

    

  • Select activity Content of the fourth axis

    

    Content of the fourth axis File

    Completion

    Students must

    

    Mark as done

    

    Dear student, Upload the file and read it well

  • Select activity Questions Axis IV : test

    

    Questions Axis IV : test Quiz

    Completion

    Students must

    

    Mark as done

    

    In case you are unable to solve exercises, you should review the lesson and reapply examples.

  • Select activity Oriented Business Series 4

    

    Oriented Business Series 4 File

    Completion

    Students must

    

    Mark as done

    

    Dear student solve the exercises in the series.

• Select topic Exit exam

  Exit exam

  

  Complete the final evaluation on time
  Important Note: The time limit for completion of the final evaluation is 45 minutes and two attempts

  Dear student in case you hide in resolving your exam by revising the lesson and applying examples

  • Select activity Math exam for El arbi Ben Mehidi University

    

    Math exam for El arbi Ben Mehidi University File

    Completion

    Students must

    

    Mark as done

    

    Dear student with a final math exam in your hands for another university, solve the exam and ensure your success rate in the right solution.

  • Select activity Exam Model Answer

    

    Exam Model Answer File

    Completion

    Students must

    

    Mark as done

    Dear student, here's the typical answer to the exam, check your answers

    

• Select topic Homework for group N° 9 & 10

  Homework for group N° 9 & 10

  

  • Select activity Homework

    

    Homework File

    Download tis file and solve the homework

  • Select activity recuperation of homework

    

    recuperation of homework Assignment

    Dear student post your homework here

• Select topic Solved previous exams

  Solved previous exams

  

   

  • Select activity Exam 1

    

    Exam 1 File
  • Select activity Exam 2

    

    Exam 2 File
  • Select activity Exam 3

    

    Exam 3 File
• Select topic reference list

  reference list

  

  The student must see all the references at his disposal in order to ensure the good way to acquire all the targeted competencies and thus the proven success. Among the references we place in the students' hands are the following:

    Mas' adan Hadi and Hanashi Farah, General Mathematics of Economists, edition 1, Noran Publishing and Distribution, Tibsa, Algeria, 2021.

  ·            Hussein Yassin Tamah, Mathematics for Economics, Administrative and Financial Sciences, T1, Dar Al Safa for Publishing and Distribution, Amman, Jordan, 2010

  ·        .Jacques Dixmier (2001), Cours de mathématiques du premier cycle 1re année– Avec exercices corrigès, Deuxième édition, Dunod, Paris, France.

  4. Jacques Vélu (2020), Mathématiques Générales - Cours et exercices corrigès, Dunod, Paris, France.
• Select topic finally

  finally

  My wishes to dear students for success, thank you.